Hyperbolic plane tilings and polyhedra_
In my new web toy you can see a sphere morph into a hyperboloid and watch the effect on a plane tiling and its projection as the curvature changes.
http://timhutton.github.io/hyperplay/projection_2D.html
Intriguingly, the hyperboloid model is also how spacetime works in special relativity (Minkowski space), so there's a deep connection between hyperbolic space tessellations and the world you're sitting in right now.
Related demos and source code are here: https://github.com/timhutton/hyperplay
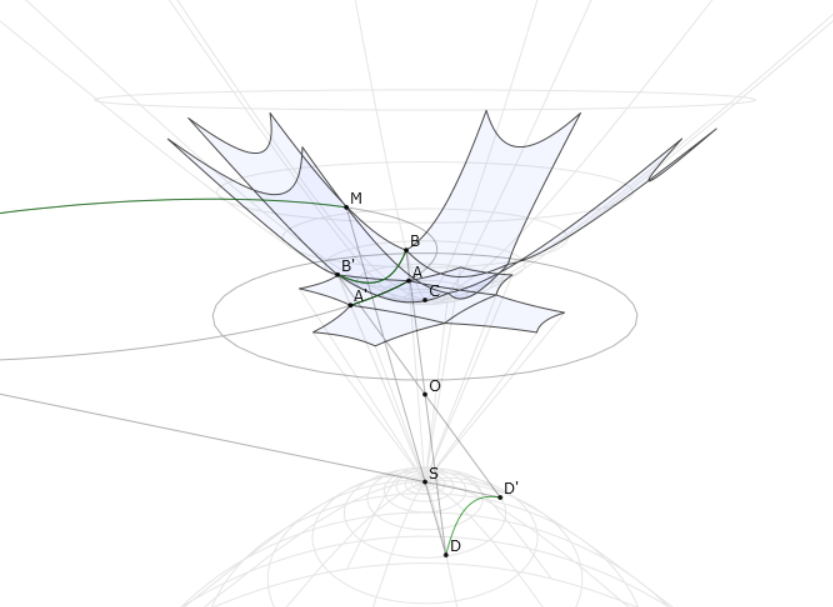
Hyperbolic plane tilings and polyhedra both lie on curved surfaces, with negative and positive curvature respectively. But how can a surface have negative curvature, when the radius of a circle is always positive? It turns out that a circle can have an imaginary radius, and hence a negative squared radius. And what does a circle with an imaginary radius look like? It's a hyperbola. That's why we call it a hyperbolic space. In my new web toy you can see a sphere morph into a hyperboloid and watch the effect on a plane tiling and its projection as the curvature changes. http://timhutton.github.io/hyperplay/projection_2D.html Intriguingly, the hyperboloid model is also how spacetime works in special relativity (Minkowski space), so there's a deep connection between hyperbolic space tessellations and the world you're sitting in right now. Related demos and source code are here: https://github.com/timhutton/hyperplay
This post was originally on Google+
